Bummer.
Tutor Coach has officially closed (July 1, 2022).
You’ve reached Adrianne Meldrum’s home base for serving students.
Explore Made for Math!
Join the Made for Math Team
We hire qualified teachers in the following US States: AZ, CO, ID, FL, ID, MO, NM, TX, UT, WA
Browse our open positions below:
MOST RECENT TOPICS
Dyscalculia Diagnosis (as an adult): Is it worth it?
Navigating the path to obtaining a dyscalculia diagnosis as an adult can be both daunting and...
Mathematics For All: Supporting Different Learning Student Profiles
At Made for Math, we understand the diverse learning profiles of our students and recognize the...
CRA Method: Concrete-Representational-Abstract–What Is It and How To Use It
Have you ever heard of the CRA Method in math instruction? It stands for Concrete,...
I NEED HELP WITH…
Number Sense
Number sense is a student’s ability to understand, relate, and connect numbers. It includes the ability to understand quantities and concepts like more and less. Here are some number sense tips to help.
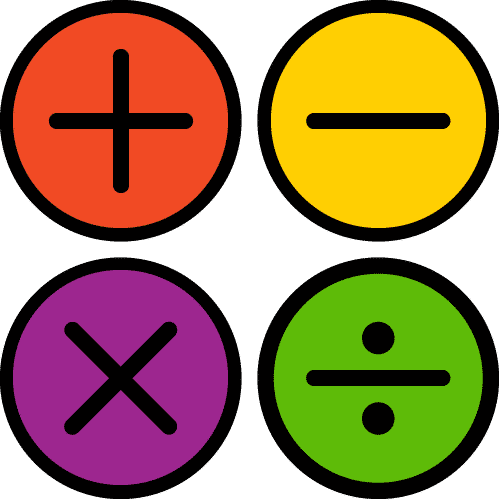
Basic Operations
Does your child struggle with basic math operation like addition, subtraction, division and multiplication? Here are great ideas to help with that.
Math Facts
Math facts seem to be a struggle for many students. You don’t have to drill the flash cards over and over. There are a lot of other more helpful ways to learn those math facts and have them stick.
Money and Time
Why is money and time so difficult to learn for a lot of students? Don’t fret, check out these awesome techniques to get your child back on track.
Fractions, Ratios, and Percent
Fractions, Ratios and Percents…these words are sure to clam up most any kid that we see. Did you know that we have fun ways to teach these? Check them out here.
Pre-Algebra/Algebra
From polynomials to intergers to graphing, we have tips to help with any of these topics. We cover a lot of Pre-Algebra and Algebra topics to help students along the way.
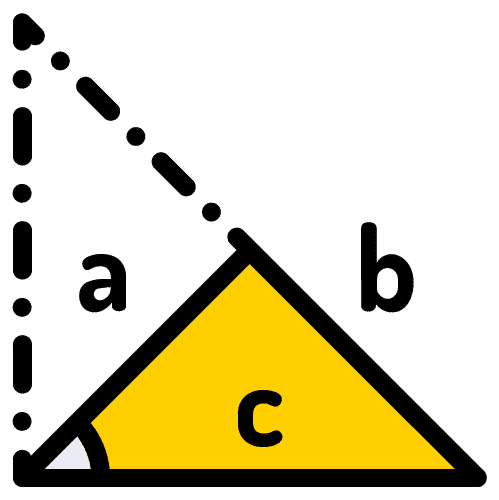
Geometry
Geometry primarily focuses on points, lines and planes. We can help guide your child through these concepts in a clear and concise way.
Trigonometry/Pre-Calculus
Yes, we even specialized techniques to help learn trigonometry and pre-calculus. Higher math like this can get tricky for students, but our techniques are sure to help!
Multisensory Math
Multisensory Math is what we focus on at MFM. It is a different way of thinking about teaching math and was developed by Marilyn Zecher. It uses objects to teach the concepts of math. This method leads to the use of pictures to provide a kind of portable memory which supports processing at the abstract level which uses only numbers. It is very beneficial in teaching children with learning differences.
Learning Differences
At MFM we believe that everyone is Made for Math! Not all brains are wired the same, and that’s ok. We primarily work with kids with Autism, ADHD, Dyscalculia, Dyslexia and other learning differences. We have a method that is highly successful in teaching these children math. We have a lot of resources availiable to you to learn more.
Webinars
We have a series of informative webinars that cover all kinds of helpful topics to help you on your journey with your child’s different way of learning. These webinars are primarily geared toward parents and offer great insight to those seeking answers in their child’s education.